a

 

Tornado-genesis by an Isentropic Energy Transformation

(June 2008)

 

 

Summary:

 

Text Box: V = [2cpΔT(ΔT/T)]1/2 (Heat engine) V = n1/2co ( ΔT/T)1/2 (Isentropic) Analysis of a very simple, rare whirlwind occurring over cool lake surfaces indicates that an isentropic transformation of heat energy into the kinetic energy of wind speed occurs.

 

The same transformation can explain the initial high wind speeds observed in tornadoes at their formation, where a heat engine explanation fails completely.

                                        

 Contents

1. Introduction

 

2. Vortex Motions

3. Is the Tornado a Heat Engine?    

4.  Waterspouts and Dust Devils as Heat Engines

 

5.  Fine Weather “Cool-lake Whirlwinds”(CLW)

 

6. An Alternative Explanation:  Isentropic, Adiabatic Flow Transformations in Vortex Flow   

 

7. A Possible New Insight into Tornado-genesis at Temperatures Inversions Aloft

 

8. Insights into Potential Tornado Modification or “ Control”

 

9. Conclusions

 

References

 

1.  Introduction

 

Tornados, waterspouts and dust devils are sometimes considered to be atmospheric heat engines, transforming heat energy differences  (Δ Q, ΔT)  between a heat source of higher temperature in the air at the earth’s surface and a heat sink region of lower temperature aloft, into kinetic energy ˝ mV2 and into wind flow V and work W.

 

However, the initial formation of these atmospheric whirlwinds in regions initially of small temperature differences means a very  small thermodynamic heat engine efficiency  and very small wind speeds, and this conflicts with the quite sudden appearance of high winds. Some theories have attempted an explanation by introducing additional sources of energy other than heat, such as lightning stokes, into the vortex core  [1,2] to account for this discrepancy.

 

Current research has concentrated, instead, on a greatly improved understanding of the many complex dynamic details of these violent storms provided by the availability of such tools as Doppler radar  to measure  internal wind speeds in the vortices, and on numerical simulation to model a wide range of flow possibilities. Once fully formed, these complicated violent storms are now fairly well understood [3] but the early stage mechanism is not.

 

Here, we shall examine a very rare, extremely simple, yet violent atmospheric whirlwind which occurs under weather conditions  which are apparently completely the opposite to those in other whirlwinds.  Tornados and waterspouts form over warm surfaces in windy cloudy weather. This rare whirlwind forms over cool lakes under fine, sunny,  calm  conditions, with the vortex  arising suddenly and violently in a very small area while all else around remains undisturbed. We shall call these special vortices  “ cool-lake whirlwinds’ ( (CLW) for want of a better name, and see if their extremely simple structure may offer clues to understanding the  more violent and complicated storms such as tornadoes and waterspouts.

 

 

 

2. Vortex Motions

 

A Vortex  involves organized circulatory or rotary motion of a fluid (i.e.  liquid or gas) in which the fluid motion is round and round about a central point or central  axis. The swirling motion of water slowly draining from a sink or wash basin is an example of a vortex. The flow  around the perimeter of the basin and in the  main body of the water in the wash basin  is circulating,  but it is not technically rotating. This seems a bit contradictory, but, for example, a floating cork which has a straight index line inscribed on its top, circulates around and round with the water, but the orientation of the line inscribed on the cork acts much as the needle of a compass and remains pointing in the same direction.. While the cork and the water it is floating in certainly do circulate, they do not themselves rotate. However in the very centre of the swirling water, if there is no actual gap in the fluid, the motion is different ---there the water core rotates in a wheel-like manner so that the rotation becomes zero at the central axis.

 

When the flow is steady, the outer, non-rotating circulation is described mathematically as

 

VT r = Γ =constant

 

were Γ with dimensions of angular momentum is called the circulation, VT is the  velocity at any point of radius r.  An example of this type of circulatory motion is the  potential vortex, often called a free vortex,  in which the motion can be described by a potential velocity function φ, such that dφ/dx = V   [4,5,6]. 

 

The  true rotational motion  in the inner  core is called solid or wheel-like rotation, or sometimes a forced  vortex or rectilinear vortex, and is described  mathematically as

 

VT/r = ω

 were ω is the rotation with the dimensions of frequency.

 

Atmospheric vortices typically consist of an outer circulatory whirl of irrotational or potential motion) enclosing a very small inner core of solid rotation.   The presence of the solid rotational core is  physically necessary because a pure  circulatory or potential vortex  would require a velocity V of  infinity at the core where r = 0, and  so, in nature, some adjustment is necessary. This problem of infinity is avoided by  a core having solid-like rotation, since then the velocity drops to zero at the central axis where r = o as required. The two flow systems 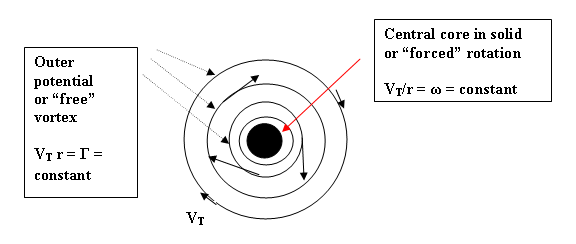together are often called a Rankine combined vortex  [4,5,7,8] .

Figure 1 Rankine Combined Vortex.

The classical work on vortex motions in fluids by Helmholtz, as extended to air motions by Rayleigh, is described by Lamb [4] and by Prandtl and Tietjens [5].  In 1934 Brunt  {9}applied this vortex theory to explain  the structure of hurricanes and tornadoes. For simplicity, Brunt assumed air to be  incompressible and  postulated the vortex structure to be a Rankine combined vortex , i.e.  a forced vortex core, surrounded by a free or potential vortex. This model was then widely used to explain these atmospheric vortices in a general way, but correspondence with the observed details of structure and dynamics was found to be insufficient. For example, to explain the high wind speeds in tornadoes in incompressible theory, a radial inflow into the vortex with conservation of angular momentum had to be postulated.  This would explain the spin-up from low speed in the outer region to high tangential wind speeds in  the core through conservation of angular momentum  ( Vr = Γ =const.) in the radial  inflow. However, with inflow, it was also necessary to then postulate an outflow ( at high levels in the atmosphere) in order to keep the process going and avoid “filling up” the vortex.  This in turn led research off into the complex details of storm cloud dynamics.

 

In 1954 Abdullah [10], in an effort to improve on Brunt, applied the theory of compressible air flow to tornadoes which then  permits the transformation of internal heat energy into kinetic energy or motion.  He postulated that the maximum speed would then be the critical or Mach 1 speed given by  V*  = [  n/n+1 ]1/2 co.  where V* is  equal to c*, the sonic speed, at Mach 1  ( approx. 301 m/s for air with n = 5). Here n is the energy partition parameter related to the ratio  of specific heats k as n = 2/ k−1, where k = cp­/cv..

  

Vonnegut [1] showed later that the Abdullah sonic velocity  limit ( M = 1) could not be reached thermodynamically via a heat engine transformation, given the poor efficiency of such engines and the  known temperature differences in the atmosphere between ground and the stratosphere ( about 70˚C versus about 115˚C needed)  and so he postulated an input of electrical energy from lighting strikes in the tornado core in order  to make the heat engine model work.   [Eventually it was determined [7, 15] by observations that  tornado speeds at the ground are actually much lower, at around   100 to 130 m/s, and  so the  energy upgrading hypothesis was not needed].

 

We now proceed to examine two compressible flow models: first the heat engine model and then an isentropic  flow model.

 

 

3.0  Is the Tornado a Heat Engine?

 

The large scale characteristics of  the tornado [3] include:  (a) Extremely strong swirling ground level wind speeds ranging from about 75 miles per hour ( 33 meters per second) in the weak category (F1), up to over 220 mph ( 100 m/s ) in severe tornadoes (F4), and to over 300 mph ( 130 m/s) in  supercell storms (F5).

   

 The earlier idea [1,2,10] that tornado wind speeds at the core were actually sonic ( i.e. about 300 m/s, 670 mph )  led to great difficulties in finding the necessary atmospheric energy source to account for such extreme wind speeds. Later observations [7,16] that the maximum boundary level  winds are only  about 130 m/s  has resulted in models that are much closer to the observations.

 

(b) The  visible, rope- like funnel  of the tornado  or ‘ twister’, is not the entire swirl of wind, but is only the visible condensed cloud of water droplets which forms when the air in the whirl has expanded and cooled in the lower pressure which prevails towards the center of the funnel until the relative humidity has reached 100% .

 

 (c) The visible  funnel cloud emerges from the base of a storm  cloud at a height of typically around one or two thousand meters,  and the funnel then  extends lower and lower towards the earth’s surface.  The tip of the funnel may not reach the ground at all, and in such cases it usually retreats back up and disappears into the parent cloud from which it emerged.  If the funnel does “ touch down” at the ground,  it suddenly broadens in diameter and the swirling winds at the ground speed up.  While the tip of the descending funnel may be only a few meters in diameter before touch down, immediately afterwards the base diameter typically becomes 50 to 100 meters across and may increase to over a kilometer in severe tornados.

 

(d) The general air mass in which the storm forms is always very convectively unstable with warm moist air at the ground and cool air aloft. The parent cloud often is already, or soon becomes, a violent thunderstorm cloud. The most severe storms are called super-cell thunderstorms.

 

A standard scale of tornado intensities devised by Fujita is shown in Table 1.

 

                    Table 1

 

Fujita Scale of Tornado Intensity

 

F  Value           Description                   Wind Speeds   

                                                                        m/s                               mph     

F0                    Light                             18-32 m/s                    40-72 mph      

F1                    Moderate                     73-112 mph                 33-50                          

F2                    Significant                     113-157                       51-70                          

F3                    Severe                          158-206                       71-92                          

F4                    Devastating                   207-260                       93-116            

F5*                  Incredible                     261-318                       117-142                

F6*                  Inconceivable               319-379                       143-170                      

                       

Note: Lewellen [ 7] writes “ maximum low level velocities are limited to less than 110-130 m/s.” This limitation solves the problem raised by earlier ideas [1,2,10] that maximum tornado winds were near sonic speed [ 300 m/s, 670 mph] . Such speeds placed impossible requirements on the  temperature differences ( ΔT ~ 115˚C) needed to explain tornados as heat engines,  and as already pointed out above, led Vonnegut  and others [1,2] to theorize about lightning strokes in the  funnel core providing an additional source of the energy needed for such extreme speeds.

 

One common explanation of a tornado has been that it is an atmospheric heat engine driven by convective instability between  a warm moist source at the ground level and the upper atmospheres at much cooler sink temperatures, the general temperature differential in a mature tornado thus being about 40 - 50°g C. The vorticity, i.e. the swirling or rotating motion is usually described as having originated  from wind shear between interacting air streams  in the storm system [3]. 

 

The heat engine is an  irreversible, non-isentropic flow, where the relationship between velocity V, and thermodynamic quantities  Q, and T,   is given [1,6] by:

 

V =  [ 2 cp ΔT (ΔT/To) ]1/2                                                                       (1)

where cp  is the specific heat at constant pressure ( 0.244  gram calories  in air at 20C), ΔT = To − Ti is the temperature drop from source to sink, and   ΔT/To  = ( ­­ To − T)/To  = 1 − T/To is the thermodynamic efficiency of the heat engine as required by the 2nd law of thermodynamics, and which limits the amount of work that can be extracted from any given heat input. ( The work done  ( dW = pdv) enters through expansion (dv)  of the air in the vortex).  Table 2 shows the values of velocity V for given values of temperature difference ΔT computed from Eqn. 1. for atmospheric air.

                                                                                   

                                           Table 2

 

Maximum calculated  wind speeds in the  heat engine model (*)

 

V = [ 2 x 0.244 x 4186 x ΔT x  eff. ]1/2  meters  per sec.; To = 293˚C

 

ΔT       T          Efficiency [ΔT/T]                      Tangential Velocity  V             

                                                                                                                                  

0.5       292.5     0.5/293= 0.0017                    1.32 m/s           3.0 mph**                               

1          292K   1/293 = 0.0034                        2.63                 5.9                              

2          291      2/293 = 0.0068                        5.3                   11.8                                        

3          290      3/293 = 0.01                            7.8                   17.5                            

4          289                  0.014                           10.7                 23.9

5          288                  0.017                           13.1                 29.5

6          287                  0.02                             15.7                 35.0

7          286                  0.024                           18.5                 41.2                

8          285                  0.027                           21.0                 47.0

9          284                  0.031                           23.9                 53.4

10        283                  0.034                           26.4                 59.0

12        281                  0.0.041                        31.7                 71.0

15        278                  0.051                           39.5                 88.4

20        273                  0.068                           52.7                 117.9

25        268                  0.085                           65.9                 147.4

30        263                  0.102                           79.1                 177

40        253                  0.137                           105.8               237                             

50        243                  0.17                             132                  295                             

70        223                  0.238                           184                  411

100      193                  0.34                             263.5               590

115      178                  0.39                             302.7               677                                                     

* cp = 0.244 cal /g/˚K= .244 x 4186 = 1021.4 Joules ;   **   meters /sec x 2.2369 = mph

 

 

 

Note 1.  Values in red are those corresponding to maximum observed   temperature differences in the troposphere, that is to maximum wind speeds attainable via the heat engine thermodynamic transformation. Values in blue correspond to the usual small temperature differences existing in the atmosphere when vortices first form.

 

 

 

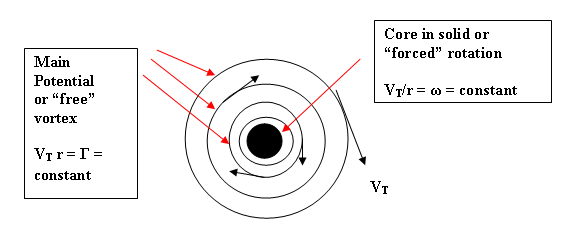
Figure 1. Rankine Combined Vortex

 

On the assumption that the flow velocities V in Table .1 apply to the tangential velocities  VT  in the vortex. (Fig.1 above )  it is clear that  even weak tornadoes with winds of say 100 mph ( 45 m/s) will require temperature contrasts of about 15 to 20 degrees C to form. These large temperature contrasts are not evident in the initial whirls or funnels that extend down from low lying storm clouds  only 2000 to 3000 feet above ground so that the heat engine fails to explain their origin.

 

The maximum tornadoes from super cells that have vertical convection paths extending from ground level  to near the stratosphere do have the temperature contrast from  bottom to top (source and sink) of say 50 degrees C, with the ground source inflow having a temperature of say 293 degrees and the cloud tops at say  240 degrees, but this is so only after the tornado cloud  is fully grown  to its greatest height. The initial  funnel reaching down from the parent cloud layer at say 2 to 3 thousand feet above ground, typically has a temperature difference  between cloud and ground ΔT of only about 5C degrees or so, and is therefore  able by itself to produce funnel winds of only 13 m/s ( 30 mph)  under the thermodynamic  heat engine restrictions of small efficiency (Table 1) . 

 

Clearly, other sources of  energy input  must be found, other than purely convective instability temperature contrasts between cloud and ground, to account for the much greater intensity of tornado winds that actually occur. We see from Table 1 that the a heat engine model with the temperature differentials of not more than 3 deg C that are available initially can only account for winds of say 7.8 m/s ( 17.5 mph) instead of the 30+  m/s . The conclusion must be, that, in its initial stages, the tornado is not a heat engine.

 

Once the funnel touches down at the earth’s surface intensification occurs. The winds increase and the funnel base broadens. The source of this additional input of energy appears related to the infiltration of warm and moist surface air into the vortex as the drag of surface friction unbalances the purely tangential flow and permits a partial radial inflow of surface air. However, even, say, 3˚C  warmer air  plus  2˚C  degrees from additional condensation of water vapour to visible funnel cloud for a  total of say , 8˚C  will yield only  21 m/s ( 47 mph) velocity versus the 50 -100 m/s observed, which would requiring a temperature differential of from 20 to 40˚C. Again the heat engine hypothesis fails even with addition of energy from condensation of water vapour.

 

We shall shortly (Section 6)  explore a different thermodynamic process than the heat engine, which does yield the observed higher wind speeds in the initial tornado stage. But first, we explore waterspouts, dust devils and a very rare, small whirlwind which occurs in fine weather over cool, calm water surfaces on lake and rivers.

 

 

4.0  Waterspouts and Dust  Devils  as Heat Engines?

 

Waterspouts [11]  have most of the characteristics of tornadoes except that they occur only over water and that they are less intense. Typically waterspouts are about the strength of a category F1 tornado with surface winds of about 75 to 100 mph. They develop from overhanging cumulus or cumulonimbus clouds and intensify as the funnel reaches the water surface. This seems to indicate that the intensification is due to (1) some surface friction  causing warm air inflow and (b) and condensation of water vapour from the moist surface layers over the water. The surface friction over water is much less than over land and so the surface air inflow and heat input from it are  much less than with tornadoes, thus pointing to  a lesser intensity .

Thus far the general features of both tornadoes and waterspouts are that they are vortices of somewhat unknown initial formation, fuelled by an infusion of  latent heat of condensation,  but with wind speeds that are much greater than can be explained by treating the  vortex as a heat engine 

 

Dust devils [12] are essentially small, rather weak, atmospheric heat engines fuelled only by hot air inflow at the surface, instead of also by condensation of water vapour. Dust devils occur under cloudless conditions over intensely heated desert or other barren surfaces.  They apparently start as random swirling eddies, and, if intense solar surface heating and air inflow are right, they intensify to whirlwinds of say 50 to 100 mph. ( 22 -45 m/s). They are visible only from the swirling dust and sand that they stir up. With only one source of heat input they are much weaker than most tornadoes.

 

To learn more about the  details of atmospheric vortices  and their inner causes we now turn to an almost unknown atmospheric whirlwind that occurs only in fine, calm, warm weather over cool waters, such as on lakes  or on calm stretches of cool rivers.

 

5.0  Fine weather “Cool- lake whirlwinds”(CLW)

 

These rare, mysterious whirlwinds first came vividly to personal  attention on Sept.2, 1987  during  a fishing expedition on a small lake in Mont Tremblant Provincial Park north of Montreal, Quebec. My wife, Pauline, and I were in a  rowboat on Lac  la Fourche, a  small body of water, about a  third of a mile long  by half that distance wide.  It was a  fine, warm sunny morning with little or no wind. At about 11 a.m. my wife suddenly called out in alarm at the sudden appearance out of nowhere  of a small, whirling, column of mist  over the water  in a small bay about 100 meters from our boat and about 50 meters from the wooded shoreline.

 

 The swirling mist column was about 2 meters feet high  and 1.5 meters in diameter, embedded in a  larger  patch of disturbed surface water about 8 meters in diameter. The mist column was whirling round and round violently and emitting a hissing sound. After about 10 to 15 seconds  in the same location  it disappeared as suddenly as it had formed, leaving only a small darkened,  breeze- ruffled area on the surface of the water which  then drifted away across the lake at about 10 km/hr toward the eastern shore. This lake is shallow, not over 7 meters or so deep at the deepest spot.

 

Inspection of  the little bay where the whirlwind  had erupted showed no signs of any disturbance in the water itself which was perfectly clear right down about a meter or so to the lake bottom. Obviously the whirl had been in the air above the water only. The lake water was cool at  around 18 ˚C. The air  temperature was warm at about 25˚C ( 24 to 26˚C). This situation, with cool air at the surface and warmer air above is just the reverse of the temperature stratification that occurs in tornados and waterspouts where the air is warm at the surface and cooler aloft.

 

Technically stated, the usual atmospheric situation in whirlwinds is  convective instability. This little lake whirlwind was, however,   completely stable, forming under an inversion of temperature and with cooler air below at the surface.  To a meteorologist this was all completely mystifying.  There was no wind to initiate any swirling or vorticity by wind shear; the sudden little vortex was violent with winds estimated at 30 to 35 m/s (68  to 78 mph), there were no storm clouds,  etc. etc.

 

Investigation showed that  there was only one other account in the scientific literature of a similar phenomena occurring  in North America. That one reported a whirl of spray “ the size of a flour barrel”  suddenly appearing on a small lake in the Adirondack   Mountains, N. Y State  on a fine sunny day in May 1920  [13]. It gave off a “splashing” sound and lasted a minute or so. In Europe there were reports of a couple of somewhat similar occurrences [ 13 ].

 

Further investigation revealed that  Bernard Bruneau, Park Warden of Mont Tremblant Provincial Park for 24 years, had also seen such  tiny whirlwinds on the Park’s lakes  two or three times during his career, and once on a calm stretch of a small river. All had occurred on fine, sunny, warm, nearly windless days.

 

Clearly what we had seen was a very rare event.  It also was so simple, meteorologically speaking, compared to tornadoes and waterspouts, that it invited close scientific analysis. The general situation is shown in Figures 2,3,4.  

 

 

 

 

 


Fig. 2. Watercolor sketch of the vorte                                          

 

 

 

 

 

 

 

 

 

 

 

Fig. 2. Watercolour sketch of small rare whirlwind seen  on Lac la Fourche,  Parc Mont Tremblant, Quebec on Sept. 2, 1987

 

 

 

18˚    23˚  25˚
 
                                   

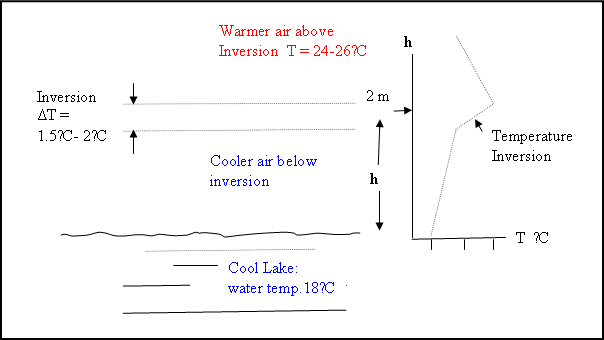
Figure 3. Temperature profile over cool lake surface

 

Cool water temp.  18˚C
 

 

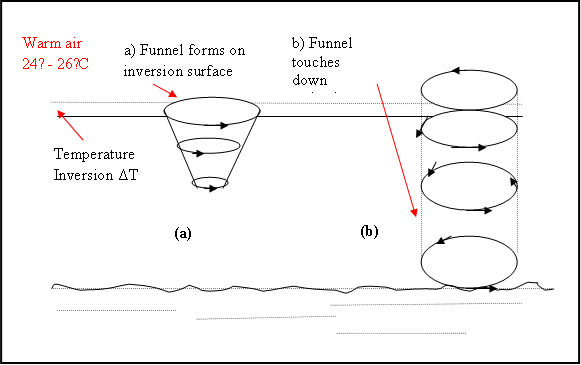
Fig. 4  Schematic cross section ( a)  Incipient whirlwind   (b) The fully formed vortex.

 

The first conclusion of the study was (1) that, if the vortex were an atmospheric heat engine, then  the heat source must be the warm air just  above the temperature inversion at a height of about a meter or so above the surface of the lake; (2) the heat difference  ΔT  between a heat source in the warm air above the inversion and the heat sink in the core of the cortex at the lake surface could not be more than the temperature difference between water temperature and air temperature which was at most  about 7˚C, and more likely was only about half that value or 3.5˚C.  In that case the resulting winds from Table 1 would be only 9 to 18.5 m/s, far below the 30 to 35 m/s observed..   We cannot therefore explain this cool-lake whirlwind  (CLW) as a heat engine transforming heat energy  into wind speed.

 

Even adding in heat of condensation from the formation of the column of mist in the vortex core will not make up the difference. The liquid water content of clouds ranges from 0.2gr.  to over 3 grams per m3 . Each gram condensed out released 680 calories of heat. Therefore the temperature rise of the air into which the vapor condenses can be calculated from the heat Q released into the air per gram of water vapour condensed to liquid which is Q = 586 calories /gm.  ( or 2.454 Joules ).

 

In Table 3 the temperature rise in one cubic meter of air by an injection of 586 calories of heat ( 2.454 Joules) is calculated from Q = mass x  ΔT x specific heat of air.  Thus, ΔT = Q / m x 0.244 = 586 / 1000 x 0.244 = 2.4 degrees per gram of water vapour  condensed to liquid cloud droplet form. Neglecting several small corrections, such as for ventilation  and so on, we get the following approximate  temperature rises to be expected from condensation: :

                                                            Table 3

 

                        Approximate Temperature Rise ΔT Expected from

                               Cloud  Condensation in a Vortex Core

 

                        Condensed Liquid water                       Temperature rise (ΔT)/m3

                                    0.5 g/m3                                                                               1.2 C

                                    1                                                                      2.4 C

                                                           

 

 

Therefore, condensation of the water vapour in  the inflow of moist air at the surface of the lake into the vortex would apparently add only 1 to  2.4 ˚C to the heat input, still  much too low  to explain winds estimatd ate 30 to 35 m/s.

 

Finally, we cannot  expect the inflowing air at the surface of the lake  to add heat, as it does with tornadoes at their touching down, since, in this case , the lake water is cool and the air over it, while moist,  would still be cool. So, any inflow of surface air would be cool instead of warm and would reduce the  vortex winds instead of increasing them..   It appears then that the heat engine model  fails to explain the sudden violence  of these tiny rare vortices and that some  other mechanism must be sought.

 

 Turning from the heat engine model, we now, instead, examine an  isentropic  transformation relating heat  energy in the form of temperature differences ΔT to the  kinetic energy  ( ˝ mV2) and flow velocity V.   Since the transformation is isentropic, no work is done, the thermodynamic efficiency restriction  of Equation 1 does not apply, and much larger velocities  emerge.

 

 

6.0  An Alternative Explanation: The Vortex as an  Isentropic, Adiabatic Flow Transformation.   

 

The isentropic transformations of  energy from heat into  flow velocity  with no work done, is given by [6]

 

V = n1/2 co [1 −  T/To]1/2 = n1/2 co [ ΔT/To]1/2                     (2)                                                                                                    

.

 

This is derived as follows; We start with the kinematic energy equation in terms of wave speed c, static wave speed  co, temperature T, flow velocity V, and n, the number of ways the energy of the air is divided. ( n also equals 2/ (k − 1)  where k is the ratio of specific heats cp/cv.  and has the value of 1.4 for air.)  As is usual in flow theory, we consider unit mass of fluid so that m  (= 1) does not appear explicitly in the equation.

 

c2 = co2   − V2 /n

c2/co2 = 1 − V2 / nco2

 

 

If we now further restrict the adiabatic case to isentropic  flow, we can apply the usual isentropic relations

 

c2/co2 =( p/po)2/n+2 = ( ρ/ ρo)2/n = T/To and so 

 

T/To = 1 − V2 /n co2 ,  whence we  have Eqn. 2 above

 

V = n1/2 co [1 −  T/To]1/2 = n1/2 co [ ΔT/To]1/2

 

which gives the relationship between flow velocity V and the temperature drop ΔT

 

Table 4 gives the  computed values of V for a range of  temperature drop ΔT ,calculated from Equation 2, and  taking  n = 5,  co = 330 m/s, and To as 293  ˚K. 

 

 

 

 

 

 

 

 

Table 4

 

Flow Velocity Versus Temperature Change in Isentropic Adiabatic Flow

V = n1/2 co [ΔT/To]1/2

 

For To = 293 K ( 20C), n = 5 , co = 330 mps

 

            ΔT                   T                      ΔT/To                                    V

-----------------------------------------------------------------------------

                                                                                    m/s                   mph

 

               0.5                  292.5             0.0017                        30.4                 68.1

               1                     292                0.0034                        43                    96.2

               2                     291                0.0068                        61                    136.4

               3                     290                0.01                            74.7                 167

               4                     289                0.014                          86.2                 192.9

               5                     288                0.017                          96.4                 215.6

              10                    283                0.034                          136                  304.2

              15                    278                0.051                          167                  373.4

              20                    273                0.068                          192.8               431.2

              40                    253                0.137                          273                  610.7

 

Note 1: Values in blue are for the observed cool lake vortex wind speeds and corresponding temperature differences. Values in red are for the maximum observed tornado wind speeds [7,16].

 

It is apparent that isentropic flow velocity transformations can account for the magnitude of the  winds arising in the CLW from very small temperature differences in the inversion over the cool lake surface.  Table 4 shows that even a temperature differential at the inversion level of only 0.5˚C transforms  or corresponds to a flow velocity of 30.4m/s or 68.1mph,  while a 1˚C temperature differential would produce  a wind speed of 43 m/s ( 96.2mph).   Therefore we can now explain the Lac de la Fourche observed wind speeds of 30 to 35 m/s from the temperature difference in the  temperature inversion over the lake with an isentropic energy transformation.

 

We must note, however, that there remain certain difficulties with the proposed isentropic explanation. Strictly speaking, such a transformation requires that there be no heat input or output. This would seem to rule out latent heat of condensation being involved, and yet the visible condensation funnel is an essential fact of these vortices.  Again, there is the fact that surface temperature inversions over cool waters are very common in the atmosphere, yet these violent little vortices are very rare.  The general rule is that atmospheric processes are non-isentropic, involving radiation heating or cooling, condensation and evaporation, and non-isentropic turbulent exchange all of which act to overmaster the isentropic process.

 

However, the sudden appearance of these rare violent vortices is a fact, and the isentropic process can explain their sudden appearance and their wind speeds. Their subsequent evolution, of course, almost certainly would appear to be either non-isentropic or only quasi-isentropic..  

 

Finally, if this mechanism acts at one temperature inversion in the atmosphere ( CLW), it must also be able to act at other similar atmospheric inversions, large or small , and so we may also have also explained the early stages of tornados and waterspouts  as well. A temperature differential of only 10˚ C--- easily reached in the lower atmosphere-- will account for the maximum observed wind speeds in tornadoes (Table 4).

 

This would be an important step towards a complete explanation. 

 

7. A possible new insight into  tornado formation at temperatures inversions aloft

 

The initial source of the tornado funnel in the lower portion of the thunderstorm storm is now addressed.

 

 It has been reported that many tornado funnels originate in association with a microburst or downburst of cool air that  takes place under a  storm cloud as a shaft of falling rain emerges from the storm cloud. [  14, 15, 16,17].   This rain shaft cools the air by evaporation and so a surge of cooler and denser air flows out from beneath the storm cloud somewhat like a miniature shallow cold front outflow ( Fig. 3).

 

Here we have a parallel  physical counterpart to the surface inversion of temperature found in our “cool lake whirlwind ’ ( CLW) , except that now the inversion is several thousand feet above ground at the top of the cool air dome (Fig. 3).  . We therefore propose that, just as the CLW vortex was generated  in the surface inversion over the lake,, so  tornado vortices may also  form at the cold dome inversion generated by falling rain from the thunderstorm cloud (Fig. 3).

 

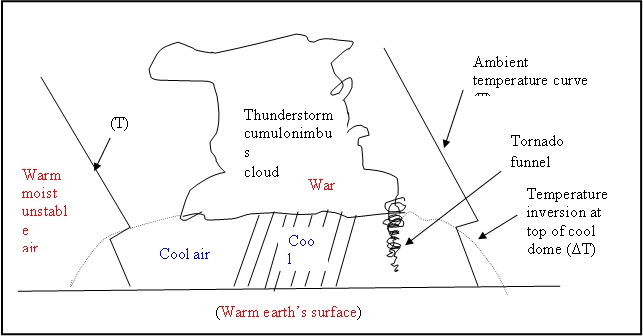
Figure. 3 Tornado forming in temperature inversion at top of precipitation-cooled air dome beneath thunderstorm cloud

 

Once the tornado funnel touches the ground, the infiltration of warm surface air would provide a second source of heat energy.  Similar considerations would apply to the case of waterspouts.

 

In this proposed model there are two key phases, first, the initial phase of the tornado prior to touchdown is not a heat engine but is an isentropic or quasi-isentropic energy transformation process (Eqn. 2), and, second, at tornado funnel touchdown a change in the mechanism from isentropic over to the non-isentropic heat engine process (Eqn. 1) begins.

 

We must point out at this point that the above new model, while it offers a solution to one key problem of the thermodynamics of atmospheric vortices, is still only one aspects of the complete picture of tornado dynamics.  The new element is a proposed solution to one current problem in all there vortices, namely the source of the initial high wind speeds.  There are, of course,  many different kinds of atmospheric vortices, gust front vortices, fair weather cumulus tornados  etc .which do not involve inversions although all involve temperature differences.   The new model also does not address the source of the initial vorticity required to start the vortex motion; this has been quite exhaustively studied and indeed appears to now be reasonably well understood [3]. The new isentropic energy transformation model proposed here, then, while it appears to solve one key problem, still requires being evaluated and integrated into the other detailed knowledge on the structure and dynamics of these great storms  If validated, it will obviously supply a key piece to the puzzle.

 

8. Insights into Potential Tornado Modification or ‘ Control’ ?

 

The new model points to the storm cloud’s temperature inversion area as the site of tornado funnel formation. Here an isentropic process takes place. Anything that interferes with the isentropic process at the inversion site would theoretically impede tornado formation. It would therefore seem useful to study this carefully from the point of view of physical and chemical changes which might permit useful intervention strategies.

 

Again, if condensation of water vapour does supply usable heat to the isentropic vortex   without disruption, then condensation inhibitors might be of interest  to tornado suppression strategies, provided that they can be employed on a sufficiently small or local scale.

 

In this latter regard, investigations into ice and condensation nucleators  some years ago       [18,19,20}] revealed that naturally occurring organic vapours, such as terpenes and other essential oil vapours released naturally from vegetation are very active in condensation processes. Ammonia vapour, on the other hand,  was found to be a powerful suppressor of condensation and of cloud droplet stability, while surfactants, such as aliphatic alcohols, and especially octal alcohol (octanol)  powerfully aided condensation and stabilized cloud droplets against evaporation. Ammonia vapour in milligram amounts produced observable effects of disrupting cloud growth and  stability at distances of  a kilometer or so downwind from the ground based points of release.

 

Examination of data on tornado occurrence and time trends of their frequency with respect to concurrent changes in agricultural crop types, agricultural practices, and environmental interventions such as pollution, might prove of  interest.   

 

 

 

9. Conclusions

Analysis of the very simple, cool lake whirlwind (CLW), points solely to an isentropic thermodynamic process as the cause.

 

The initial stages of tornadoes and waterspouts can not be described as being those of a thermodynamic or heat engine; this is because the initial atmospheric temperature differences available are much  too small and the observed initial wind speeds are far too high.

 

An isentropic energy transformation model does fit the initial temperature and wind speed data of tornadoes and waterspouts; however, any ordinary inflow of heat would contradict the isentropic process, so that a purely internal energy transformation must also be postulated. This also requires an internal isentropic flow for any latent heat released from condensation of water vapour in the incipient tornado funnel.

 

We therefore conclude that the isentropic process does play a key role in tornado-genesis, but that the details of the flow mechanism remain to be understood.

 

References

 

1.  Vonnegut, Bernard,  Electrical Theory of Tornadoes.  J. of Geophysical Re., 65, 1, pp 203 -212,  Jan. 1966.

 

2. Colgate, Sterling A., Tornadoes: Mechanism and Control. Science 157, 1431 -1434, 22 Sept. 1967.

3. The Tornado: Its Structure, Dynamics, Prediction and Hazards. Church, C., Burgess, D., Doswell, C., Davies-Jones, R., (eds.). AGU  Monograph 79, American Geophysical Union.  Washington, D.C. 1993.

 

4.  Lamb, Sir Horace. Hydrodynamics. Dover Publications Inc. New York, 1931.

 

5. Prandtl, L. and  O. G. Tietjens,  Applied Hydro- and Aeromechanics,  Dover Publications Inc., New York, 1957.

 

6. Shapiro, A.H., The Dynamics and Thermodynamics of Compressible Fluid Flow.  2 Vols.,  Wiley and Sons, New York, N.Y. 1953.

7. Lewellen, W.S., Tornado Vortex Theory:  AGU  Monograph 79, (Ref. 3 above ) pp 19-39 , 1993.

 

8. Rankine, W. J.,  A Manual of Applied Mechanics, , Charles Griffin, London, 1882. 

 

9. Brunt, David, Physical and Dynamical Meteorology. Cambridge University Press, 1941.

 

10. Abdullah, A.J., Some Aspects of the Dynamics of Tornadoes.  Monthly  Weather Review, 83, 4, pp. 83-94,  April 1955.

 

11. Golden, J, H., The life-cycle of Florida Keys’ waterspouts, J. Appl. Meteorol., 13 667-692 and 693-709, 1974. 

 

12. Idso, Sherwood, B., Tornado or Dust Devil: The Enigma of Desert Whirlwinds.  American Scientist,  62, 530-541, Sept. – Oct. 1974.

 

13. Rossman, Fritz O., Waterspouts and Tornadoes, Weather, pp. 104-106,  March 1956.  Royal Meteorol. Soc., London [ Monthly Weather Review   48, p.351 1920 ( re the May 16, 1920, re Observations on Lake Newcombe,  Adirondack Mountains , N.Y.]. 

 

14. Purdom, James, F.W.,  Satellite Observations of Tornado Thunderstorms. AGU  Monograph 79, (Ref. 3 above)  pp 265-274. 1993.

 

15. Fujita, T.T., and B.E. Smith, Aerial Survey and Photography of Tornadoes and Microburst Damage. AGU Monograph 79, ( Ref. 3 above)  pp 479 – 493,  1993.

 

16. Bluestein, Howard A. and Joseph H. Golden, A Review of Tornado Observations. AGU Monograph 79, ( Ref.  3 above) pp. 319 -352. 1993.

 

17. Davies-Jones, Robert and Harold Brooks, Mesocyclogenesis From a Theoretical Perspective. AGU Monograph 79, ( Ref. 3 above)  pp. 105 – 114. 1993.

 

18.  B. A. Power and R.F. Power,  Some Amino Acids  as Ice Nucleators.  Nature, Vol. 184, 1170. 1962.

19. -------------------------------------,  Vanillin, cis- Terpin and cis- Terpin Hydrate as Ice Nucleators.  Science. Vol. 148, 1088, 21 May, 1965.

 

20.---------------------------------------, Work on condensation nucleation and especially on organic nucleators, unfortunately had to be terminated without publication.

 

 

  Copyright 2008.  Bernard A. Power, Consulting Meteorologist ( ret.)

 

 

 

BACK TO MAIN PAGE